If a Rational Function Is Proper Then

A rational function is any function which can be written as the ratio of two polynomial functions where the denominator is not 0 0. Qx and rx can be computed using polynomial division of fxbygx.

2014 2015 Algebra 1 Unit 1 Interactive Notebook Pages Teaching Algebra Math Interactive Notebook High School Math Activities

F 1 C is compact for all compact sets C C.

. If degree of fx is greater than or equal to degree of gx then frac fx gx is called an improper rational function. Consists of the real zeros of the denominator. Step 1Use long division if you have an improper rational function the degree of the numerator than the degree of the denominator.

We discuss it here since this is a useful tool in finding integrals of rational functions but the technique can be used inside or outside an integral. Px and Qx are polynomials and. Given the reciprocal squared function that is shifted right 3 units and down 4 units write this as a rational function.

A rational function which is not proper is improperIffxgx is improper then there are polynomials qx and rx such that fxgxqxrxgx where rxgx is a proper rational function. However there is a nice fact about rational functions that we can use here. C A C be analytic and proper where A is a finite point set in C.

Neither the coefficients of the polynomials nor the values taken by the function are necessarily rational numbers. The graph of a rational function may intersect a vertical asymptote. If a rational function is proper then _____ is a horizontal asymptote.

And its proper and proper is going to mean that the degree of the denominator is larger than the degree of the numerator than this graph. If on the other hand n m then R x may be represented as a sum of a polynomial M x of degree η - m and a proper rational function R1 x P1 x Q x. Determine whether each of the following statements is true or false and explain why.

We have a rational fractions. Vertical asymptote a vertical line that a graph approaches but never crosses. That is if r is a zero of the denominator of a rational function Rx pxqx in lowest terms then R will have the vertical asymptote x r.

Gx x2 17x 12 x2 x 7 g x - x 2 - 17 x - 12 x 2 x - 7. F x P x Qx f x P x Q x where P P and Q Q are polynomial functions of x x and Qx 0 Q x 0. Profo Recall that if you divide a polynomial by a divisor then.

F z p z q z for complex polynomials p z and q z such that f. The vertical ascent oats come when Q of X is equal to zero. If a rational function is proper then _____ is a horizontal asymptote.

Many real-world problems require us to find the ratio of two polynomial functions. In other words to determine if a rational function is ever zero all that we need to do. Px Qx a polynomial Rx Qx If you have a proper rational function the degree of the numerator than the degree of the denom-inator then you are ready for Step 2.

And so when Q of X is equal to zero were gonna have P of X divided by zero or an undefined expression. You can find oblique asymptotes using polynomial division where the quotient is. Integrals of the form.

If Rx pxqx is a rational function and if p and q have no common factors then R is in lowest terms. So the zeros of the polynomial Q of X. The method of partial fractions is a general method to simplify rational functions quotients of polynomials.

Show activity on this post. So if we have this rational expression P of X divided by Q of X. In the first example the numerator is a second-degree polynomial and the denominator is a third-degree polynomial so the rational is proper.

Every rational function has either a vertical or a horizontal asymptote. If in equation 1 n m m 0 then the rational function is said to be proper. This question does not show any research effort.

Gx x2 17x12 x2 x7 g x - x 2 - 17 x - 12 x 2. For example look at these three rational expressions. Then fxgx is called a proper rational function.

If a proper rational function has double real poles 336Xs N s s α 2 a b s α s α 2 a s α 2 b s α then its inverse is 337xt ate αt be αtut where a can be computed as a Xss α2 s α and after replacing it b is found by computing Xs0 for a. If a rational function is proper then y0 is a horizontal asymptote. Long division of polynomials.

For a rational function R if the degree of the numerator is less than the degree of the denominator then R is Proper The graph of a rational function may intersect a. It is unclear or not useful. A rational function will be zero at a particular value of x only if the numerator is zero at that x and the denominator isnt zero at that x.

Qx will be the. If degree of fx is less than degree of gxthen is called a proper rational function. Proper rational functions A proper rational function is one in which the degree of the numerator is less than the degree of the denominator.

So we have some type of all set up that we have a polynomial on top and polynomial on the bottom. If the degree of the numerator of a rational function equals the degree of the denominator then the ration of the leading coefficients gives rise to the horizontal asymptote. Otherwise it is called improper.

A proper rational function is a ratio of functions where the degree of the numerator the top number in a fraction is less than the degree of the denominator the bottom number. Then find the x and y-intercepts. A rational function Rx pxqx in lowest terms will have a vertical asymptote x r if x - r is a factor of the denominator q.

Any rational function can be written as the sum of a polynomial and a proper rational function. A rational function is a function that can be written as the quotient of two polynomial functions. The degree of Px is less than the degree of Qx.

A rational expression is proper if the degree of the numerator is less than the degree of the denominator and improper otherwise. The rational function fx Px Qx in lowest terms has an oblique asymptote if the degree of the numerator Px is exactly one greater than the degree of the denominator Qx. The function has the form.

For rational functions this may seem like a mess to deal with. Any function of one variable x x is called a rational function if and only if it can be written in the form. What is a Proper Rational Function.

Note that every polynomial function is a rational. Is it true that f is a rational function ie. R x M x R1 x.

As X gets very big in the positive direction or ex gets very small in the negative direction will get. Give us the rational functions vertical assam totes.

Cbse Class 12 Maths Notes Indefinite Integrals Class 12 Maths Math Notes 12th Maths

End Behavior Of Rational Functions Video Khan Academy

How To Determine The End Behavior Of A Rational Function Algebra Study Com

Do You Know These Prefixes Worksheet Free Esl Printable Worksheets Made By Teachers Prefixes Prefix Worksheet Prefixes And Suffixes

Operations With Rational Expressions Crossword Puzzle Rational Expressions Crossword Puzzle Crossword

Partial Fractions A Plus Topper

Rational Function Graph Domain Range Asymptotes

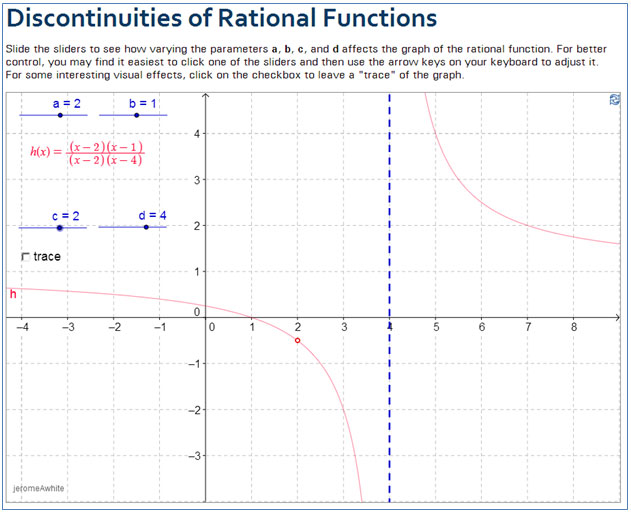
Rational Functions Predicting The Effects Of Parameter Changes Texas Gateway

Plus Two Maths Notes Chapter 7 Integrals A Plus Topper Math Notes Math Tutorials Math Formula Chart

In This Maze Students Will Try To Find Their Way From Beginning To End By Solving Integer Addition Problems An Answer K Integer Maze Integers Adding Integers